Main Index Mathematical Analysis Inequalities
 Subject Index
comment on the page

Pythagorean means

There are three Pythagorean means:

The case typeset structure

For typeset structure we have

A(a _ 1, a _ 2) = (a _ 1 + a _ 2)/2,      G(a _ 1, a _ 2) = (a _ 1 a _ 2)^(1/2),      H(a _ 1, a _ 2) = (2 a _ 1 a _ 2)/(a _ 1 + a _ 2),

and typeset structure.  Note that typeset structure is the arithmetic mean of typeset structure and typeset structure. For instance,  every term typeset structure, typeset structure, of the harmonic series typeset structure  is the harmonic mean of the neighboring terms.

Pappus [2] , Book III (12-16, p. 70-83) defines these means [1] in modern notation as follows:

Geometric mean typeset structure of two numbers typeset structure is also called mean proportional because it can expressed as the means of a proportion typeset structure.  Its construction is described in Euclid’s Elements. Proposition VI.13 of Book VI requires: To given straight lines to find a mean proportional. The construction goes as follows. Place two given segments typeset structure and typeset structure in a straight line and draw the semicircle with diameter typeset structure. Let typeset structure be the perpendicular to typeset structure. Then using Proposition VI.8 2 one gets typeset structure.

[Graphics:HTMLFiles/PythagoreanMeans_41.gif]

This gives the following geometric construction for the arithmetic and geometric means and the geometric proof that typeset structure.

[Graphics:HTMLFiles/PythagoreanMeans_49.gif]

Pappus gives the following construction: the length of typeset structure is the geometric mean of lengths typeset structure and typeset structure.

[Graphics:HTMLFiles/PythagoreanMeans_53.gif]

In  Book III he gives the following construction for the harmonic mean: Given two segments typeset structure, typeset structure, typeset structure, on a line mark off typeset structure on the perpendicular to typeset structure at typeset structure. Let typeset structure be the intersection of typeset structure with the perpendicular to typeset structure at typeset structure. Draw typeset structure to cut typeset structure in typeset structure. Then typeset structure is the harmonic mean of the given segments typeset structure and typeset structure.

[Graphics:HTMLFiles/PythagoreanMeans_73.gif]

Geometric construction of all three Pythagorean means can also be found in [3] , Book III, p. 51: Take a semi-circle of centre typeset structure and diameter typeset structure. Let typeset structure and typeset structure. Then  typeset structure. Construct the right angle typeset structure. Then typeset structure. Since the shortest distance is the perpendicular distance, typeset structure, with equality only if typeset structure. Clearly typeset structure. If typeset structure is perpendicular to typeset structure, then typeset structure. By the same argument typeset structure with equality if and only if typeset structure.

[Graphics:HTMLFiles/PythagoreanMeans_92.gif]

Another simple construction is [4], p.38:   Let typeset structure be trapezium be with two parallel sides typeset structure and typeset structure.  Then

The general case

In general we have

A(a _ 1, a _ 2, ..., a _ n) >= G(a _ 1, a _ 2, ..., a _ n) >= H(a _ 1, a _ 2, ..., a _ n)

with equality holding if and only if  typeset structure.

Clearly,

A(a, a, ..., a) = G(a, a, ..., a) = H(a, a, ..., a) = a,

and the means are homogeneous, that is for typeset structure we have

A(b a _ 1, b a _ 2, ..., b a _ n) = b A(a _ 1, a _ 2, ..., a _ n),    G(b a _ 1, b a ... _ 2, ..., a _ n),    H(b a _ 1, b a _ 2, ..., b a _ n) = b H(a _ 1, a _ 2, ..., a _ n) .

Combination of means

If typeset structure then typeset structure. If we define typeset structure, then it follows that the iterations typeset structureconverge to a single number called arithmetic-geometric mean of typeset structure. Formally, the arithmetic-geometric mean iteration is defined by the following recursion: Let typeset structure and

a _ (n + 1) = (a _ n + b _ n)/2,       b _ (n + 1) = (a _ n b _ n)^(1/2) .

Then typeset structure and typeset structure. This shows that typeset structure and typeset structure converge to a common limit typeset structure.

We also have

AG(a, b) = AG((a + b)/2, (a b)^(1/2))       or     tha ... nbsp;      AG(1, b) = (1 + b)/2 AG(1, (2 b^(1/2))/(1 + b)) .   

We can also define the harmonic-geometric mean iteration by

a _ (n + 1) = 2/(1/a _ n + 1/b _ n) = (2 a _ n b _ n)/(a _ n + b _ n),       b _ (n + 1) = (a _ n b _ n)^(1/2) .

If typeset structure then this iteration converges and

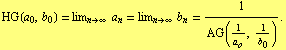
HG(a _ 0, b _ 0) = lim _ (n -> ∞) a _ n = lim _ (n -> ∞) b _ n = 1/AG(1/a _ o, 1/b _ 0) .

Finally, the arithmetic-harmonic mean iteration is defined by

a _ (n + 1) = (a _ n + b _ n)/2,    b _ (n + 1) = 2/(1/a _ n + 1/b _ n) = (2 a _ n b _ n)/(a _ n + b _ n) .

If typeset structure then this iteration converges to the geometric mean of typeset structure and typeset structure, i.e.

AH(a _ 0, b _ 0) = lim _ (n -> ∞) a _ n = lim _ (n -> ∞) b _ n = (a _ o b _ 0)^(1/2) .

Notes

1 The name harmonic mean was introduced by Hippas of Metapont (ca. 450 BC), cf. [1] ,p. 243.

2 If in a right-angled triangle a perpendicular is drawn from the right angle to the base, the triangles adjoining the perpendicular are similar both to the whole and one another.

References

[1]  Gericke, H. (2004). Mathematik in Antike, Orient und Abendland. Wiesbaden: Fourier Verlag GmBH.

[2]  Pappi Alexandrini. (1875-1878). Collectiones quae supersunt (ed. F. Hultsch) 3 Vols.. Berlin (reprint Amsterdam: Hakkert 1965).

[3]  Pappus d’Alexandrie. (1933). La Collection Mathématique (French transl. and comments  P. ver Ecke). Paris: Brügge.

[4]  Bullen, P. S., Mitrinović, D. S., & Vasić, P. M. (1988). Means and Their Inequalities. Dordrecht, Boston, Lancaster, Tokyo: D.Reidel a Publishing Company.

Cite this web-page as:

Štefan Porubský: Pythagorean Means.

Page created  .